Puzzle of the Week #527 - Allesley Park part 3
We saw last week how the paths of Allesley Park are equivalent to the edges of a truncated tetrahedron.
We also saw that a path with certain restrictions was impossible for that network of paths. Let’s give that set of restrictions a name:
A Hamiltonian Cycle is one where you visit every node exactly once and return to where you started. You don’t have to use every edge.
A Eulerian Cycle is one where you must use every edge exactly once and return to where you started, but you are permitted to revisit nodes. Such a cycle is possible if and only if each node in the network has an even number of edges.
A Linean Cycle is one where you must use every edge at least once and return to where you started. You can select a minimal subset of edges to traverse a second time, but you must do so in the opposite direction to how you traversed it the first time, and also not immediately after the first time you traversed it.
We saw how a Linean cycle is not possible for the truncated tetrahedron. In fact I’ve yet to find an Archimedean solid for which it is possible, and have proved it impossible for a couple of other shapes.
Of the platonic solids (the tetrahedron, cube, octahedron, dodecahedron and icosahedron) a Linean cycle is possible for all but one of them. The octahedron is trivially possible, as it has an even number of edges at each node.
For which one of the other four is it not possible to form a Linean cycle?
Puzzle of the Week #526 - Allesley Park part 2
We saw before how we can run the entire network of paths in 2.24 miles, by repeating the segments AC, BL, JK, HI, FG and DE. There are of course many ways of doing this.
Now I want to add extra restrictions:
If you run a segment you can’t immediately double-back and run the same segment the other way, eg you can’t go from A to C and immediately back to A. And because we are trying to form a complete cycle, you also can’t start and end on the same segment.
If you run one of the repeated segments twice in the same direction, you get penalty points equal to the distance between them, eg if you ran from D to E and later in the run ran again from D to E, you would get 14 penalty points for that segment, whereas if instead you ran D to E and later (but not immediately) ran from E to D you would not get any penalty points.
What is the fewest penalty points you can get away with?
Puzzle of the Week #525 - Allesley Park part 1
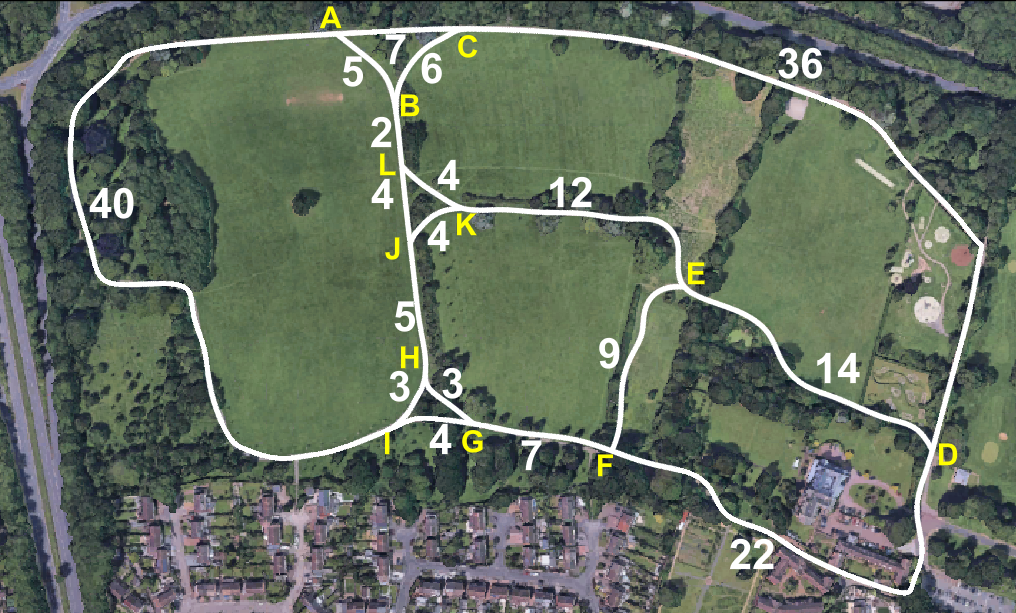
I wish to run a route that covers all of the white paths as shown, and returns to where I started. I have usefully marked all the distances between path junction points (in that well-known unit of centi-miles).
Assuming I stick to the paths, what is the length of the shortest route I can do it in?
Puzzle of the Week #524 - Four Areas
Two straight lines divide this triangle into four regions. The area of thee of those regions is given, what is the area of the fourth?
Puzzle of the Week #523 - Equal Sequences
These two geometric sequences have the same sum:
5+20+80 = 7+14+28+56 = 105
We can categorise each sequence by three parameters: the starting number, the common ratio and the number of terms. So the above sequences would be [5,4,3] and [7,2,4] respectively. For the purposes of this puzzle the parameters are all positive integers, the common ratio must be at least 2 and the number of terms must be at least 3.
Part 1: Can you find the smallest example of two sequences having the same sum?
Part 2: For the above sequences the number 4 appears twice in the parameters, as the common ratio of the first sequence and as the number of terms in the second sequence. Can you find the smallest example of two sequences having the same sum but where all six parameters are distinct?
Puzzle of the Week #522 - Geometric Sequence
The sum of an geometric sequence with n terms, the first of which is 1, is 127:
1 + r + r^2 … + r^(n-1) + r^n = 127
A subsequence that includes only every other term, but still starts at 1 and finishes at r^n totals 85:
1 + r^2 + r^4 … + r^(n-2) + r^n = 85
What is the sum of a subsequence that includes only every third term, but still starts at 1 and finishes at r^n:
1 + r^3 + r^6 … + r^(n-3) + r^n = S
Puzzle of the Week #521 - Arithmetic Sequence
S is the sum of an arithmetic sequence with n terms:
x_1 + x_2 + x_3 + … x_n-1 + x_n = S
A subsequence that includes only every other term, but still starts at x_1 and finishes at x_n totals 88:
x_1 + x_3 + x_5 + … x_n-2 + x_n = 88
A subsequence that includes only every third term, but still starts at x_1 and finishes at x_n totals 60:
x_1 + x_4 + x_7 + … x_n-3 + x_n = 60
What is the sum of the whole sequence S?
For bonus points if the first term is 0, what is the common difference between consecutive terms?
Puzzle of the Week #520 - Irregular Octagon
I have a circle of radius 19, which sits inside of an octagon, which has equal sides but not equal angles. Each of the sides of the octagon has length 17, and each of the sides is tangent to the circle.
What is the area of the octagon?
Puzzle of the Week #519 - Word Pairs
In each couplet, the second answer is the same as the first except for the addition of one letter. If you collect the five extra letters you will spell a word.
A metal red or man in blue,
A blade that whirs or cuts in two.
A spring in stride, a triple move,
A dream you have, which time must prove.
Make it round, add pounds to excess,
Or squash it down with a heavy press.
Launch with lips, a sharp eject,
Or break apart and disconnect.
A scheme to mask premeditation,
Shake to wake from meditation.
Puzzle of the Week #518 - Four Rectangles
Four rectangles are arranged as in the figure, where at every circled point the corner of one rectangle coincides with the side of another. What is the height of the magenta rectangle?
Puzzle of the Week #517 - Friday April 18th
I’d intended to publish this a few weeks ago but somehow forgot.
Why is Friday April 18th special, and why is Wednesday September 23rd equally special?
Puzzle of the Week #516 - Two Pairs of Circles
In a particular right-angled triangle, two circles tangent to the long leg have a radius or 5, and two circles tangent to the short leg have a radius of 4.
What is the radius of the incircle?
Puzzle of the Week #515 - Three Circles in a Trapezoid
Three circles are positioned inside the trapezoid below, such that they are tangent to the straight lines (but not tangent to the other circles). Given the two lengths confirmed in the diagram, what is the overall height of the trapezoid?
Puzzle of the Week #514 - Two More Circles in Trapezoids
In this shape, two circles are inscribed in two right trapezoids. The acute angles of the trapezoids add to 90 degrees. What is the overall height of the shape?
Puzzle of the Week #513 - Two Circles in a Trapezoid
Given this construction below, where two circles are tangent to the straight lines in the diagram, if we are told that A+C = B+D what is the value of A/B?
There are two possible answers.
Puzzle of the Week #512 - 4x4x4 Cube
You might have heard of the puzzle of slicing a 3cm x 3cm x 3cm cube into 27 1cm cubes, and how, even if you are allowed to rearrange the pieces between cuts, it still takes a minimum of six cuts to perform this action. There is a very clever argument that proves it.
Now consider a 4cm x 4cm x 4cm cube, cut into 64 1cm cubes. If you aren’t allowed to rearrange the pieces, will take nine cuts as shown below.
The question is: in the 4x4x4 case, with how few cuts can we slice it into 64 cm cubes if we ARE allowed to rearrange the pieces between cuts?
Puzzle of the Week #511 - Seven Circles
A triangle has an incircle of radius 5.
If two identical circles are placed within the triangle such that they are both tangent to the base, tangent to each other and each tangent to one of the other sides of the triangle, those circles have a radius of 4.
If seven identical circles are placed on the base of the triangle, all tangent to one another in a chain, and the first and last circles tangent to the other sides of the triangle, what is the radius of those circles?
As a bonus question, how many circles of radius 1 can you fit in a tangent chain along the baseline within the triangle?
Puzzle of the Week #510 - Pair of Circles 2
This is more difficult that the previous puzzle, but could benefit from insights learnt from solving that puzzle.
Given a triangle with sides 13, 14 and 15, and a pair of non-overlapping identical circles within the triangle, what is the maximum radius those circles could be?
Puzzle of the Week #509 - Pair of Circles
Given a triangle with sides 21, 28 and 35, and a pair of non-overlapping identical circles within the triangle, what is the maximum radius those circles could be?